 |
Reported below are the first sixteen harmonics of two harmonics series starting from C2 and G2, respectively. If f is the frequency of the fundamental, the harmonic series is simply f, 2f, 3f, 4f, 5f, 6f ... . Octaves correspond to a geometric series: f, 2f, 4f, 8f, ... Fundamental frequency x (Fibonacci number +1), rounded to the nearest tempered tuning pitch. No other pitch is picked by the series until the 15th to 17th terms. The Fibonicci numbers 377, 610, and 987, pick another augmented triad: G, B, D-sharp. If 0 is omitted from the series, and the rule to pick pitches is: Fundamental frequency x Fibonacci number, then the first 16 terms (1 1 2 3 5 8 13 21 34 55 89 144 233 377 610 987) pick the 12 tempered tones, with only C and G repeated. Fundamental frequency x (Fibonacci number), rounded to the nearest tempered tuning pitch Absolute frequencies are well above audible range, and for all mappings the rounding off to tempered pitch is quite pronounced.
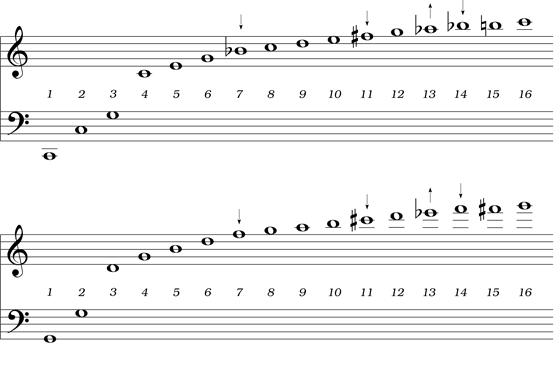
As a curiosity a mapping of the first 14 elements of the Fibonacci series (0 1 1 2 3 5 8 13 21 34 55 89 144 233) in frequency domain picks only the elements of the Luminous Chord: C G (producing a sequence of perfect fifths and fourths), D, B-flat, and F-sharp (i.e. an augmented triad),  :
:

 |
||
|